
Paris-Saclay University
Biography
I am an assistant professor in the Theory group of the Laboratory of Solid State Physics of the University Paris-Saclay. My main research interests are ab-initio theories of condensed matter, topological states of matter, and strongly correlated electron systems.
Interests
- Ab-initio theories of condensed matter
- Topological states of matter
- Strongly correlated electron systems
Education
- PhD in Physics, University of Cambridge (2016)
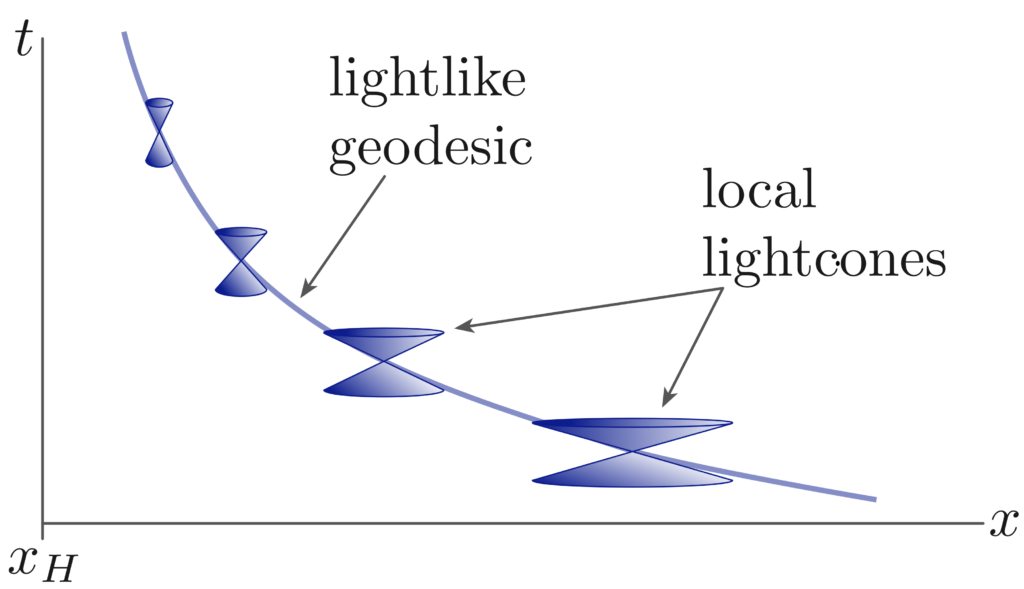
Gravitational analogues
We designed low-dimensional electronic models, related to Weyl semimetals, which mimic some of the properties of gravitational systems. In particular, we studied the dynamics of wave packets of electrons in 1D and 2D lattice models, the spectral properties of these models, and ground-state thermalisation near a gravitational horizon.
Publications
- V. Könye, L. Mertens, C. Morice, D. Chernyavsky, A. G. Moghaddam, J. van Wezel and J. van den Brink, Phys. Rev. B 107, L201406 (2023).
- L. Mertens, A. G. Moghaddam, D. Chernyavsky, C. Morice, J. van den Brink and J. van Wezel, Phys. Rev. Research 4, 043084 (2022).
- V. Könye, C. Morice, D. Chernyavsky, A. G. Moghaddam, J. van den Brink and J. van Wezel, Phys. Rev. Research 4, 033237 (2022).
- C. Morice, D. Chernyavsky, J. van Wezel, J. van den Brink and A. Moghaddam, SciPost Phys. Core 5, 042 (2022).
- A. G. Moghaddam, D. Chernyavsky, C. Morice, J. van Wezel, and J. van den Brink, SciPost Phys. 11, 109 (2021).
- C. Morice, A. G. Moghaddam, D. Chernyavsky, J. van Wezel, and J. van den Brink, Phys. Rev. Res. Lett. 3, L022022 (2021).
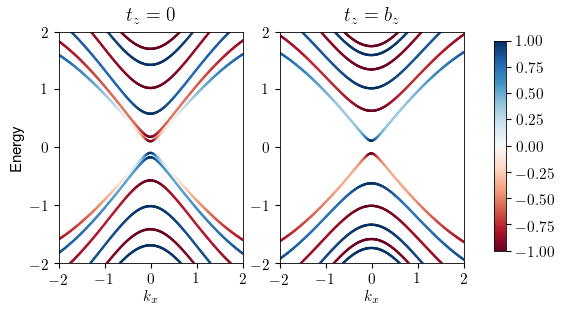
Finite-size topological insulators
We investigate the influence of finite thickness on properties of topological insulators.
We studied a minimal model for topological insulators in a slab geometry. One parameter of our model, namely the Dirac velocity perpendicular to the slab, distinguishes between two qualitatively distinct situations: when it is zero, we find that the topological invariants of the 3D system can be entirely deduced from properties of the slab. However when it is not zero, a new phase arises, with surface states but without band inversion. We also investigated this model for large dispersions perpendicularly to the slab and unveiled a regime where the slab displays non-trivial topological invariants but nonetheless extrapolates towards a trivial 3D state.
The new phase which displays surface states without band inversion is localised close to topological phase transitions. We therefore studied ZrTe5, which was shown to be a strong topological insulator, on the border of a weak topological insulating phase. We derived a model for this material fitted to ab-initio calculations and showed that it matches many experimental signatures, including optical, transport, and spectroscopic results.
Publications
- C. Morice, E. Lettl, T. Kopp, and A. P. Kampf, Phys. Rev. B 102, 155138 (2020).
- C. Morice, T. Kopp, and A. P. Kampf, Phys. Rev. B 100, 235427 (2019).
Collaborations with experimentalists
I collaborate with experimental teams performing optical measurements on very diverse materials, such as compounds exhibiting charge density wave phases. Using density functional theory (DFT) and many-body perturbation theory, in the framework of the GW approximation, we calculate observables which enhance our understanding of the measured physics.
Publication
- S. D. C. Roscam Abbing, F. Campi, B. de Keijzer, C. Morice, Z.-Y. Zhang, M. L. S. van der Geest, P. M. Kraus, arXiv:2209.15561.
- J. Groefsema, X. Feng, C. Morice, Y. Huang, E. van Heumen, Phys. Rev. Materials 6, 115402 (2022).
- B. van der Linden, T. Hogenelst, R. Bliem, K. Dohnalová and C. Morice, J. Phys.: Condens. Matter 34, 425403 (2022).
- X.Feng, J. Henke, C. Morice, C. J. Sayers, E. Da Como, J. van Wezel and E. van Heumen, Phys. Rev. B 104, 165134 (2021).
I teach physics in various tracks of the University Paris-Saclay.
Lectures
Numerical Physics — License Double Diplôme Maths-Physique (BSc, 2nd year)
Exercises classes
Quantum mechanics — CentraleSupélec (BSc, 3rd year)
Statistical Physics — Magistère de Physique Fondamentale (BSc, 3rd year)
Classical Mechanics — Magistère de Physique Fondamentale (BSc, 3rd year)
Practicals
Numerical Physics — ENS Paris-Saclay (BSc, 3rd year)
Density Functional Theory — Master in Materials Sciences (MSc, 2nd year)
Contact
corentin.morice@universite-paris-saclay.fr
+33 (0) 1 69 15 45 73
1 rue Nicolas Appert, Bâtiment 510, 91405 Orsay Cedex, France
Exercises classes
Exercise classes for the Statistical Physics course of the 3rd year of the Fundamental Physics bachelor in the University Paris-Saclay.
Contact
- corentin.morice@universite-paris-saclay.fr
- +33 (0) 1 69 15 45 73
- 1 rue Nicolas Appert, Bâtiment 510, 91405 Orsay Cedex, France
- How to reach the LPS
Contact
- corentin.morice@universite-paris-saclay.fr
- +33 (0) 1 69 15 45 73
- 1 rue Nicolas Appert, Bâtiment 510, 91405 Orsay Cedex, France
- How to reach the LPS
Contact
- corentin.morice@universite-paris-saclay.fr
- +33 (0) 1 69 15 45 73
- 1 rue Nicolas Appert, Bâtiment 510, 91405 Orsay Cedex, France
- How to reach the LPS
Contact
- corentin.morice@universite-paris-saclay.fr
- +33 (0) 1 69 15 45 73
- 1 rue Nicolas Appert, Bâtiment 510, 91405 Orsay Cedex, France
- How to reach the LPS