There are different methods, which generate quasiperiodic tilings. In a recent study, researchers from the MATRIX team propose a new method based on grids of regular tessellations of the plane that makes a bridge between methods derived from projections of high dimension lattices and methods related to Moiré patterns.
Advantage of this grid method is that it is done directly in the plane. It contains only two simple steps. First the overlapping domains and the set of their reference points is build. Then the quasiperiodic tiling is obtained from the Delaunay triangulation of this set of points, after removing extra edges. The tessellation of the plane by the overlap domains of two hexagons, each belonging to one of the two grids is considered. Vertices of the quasiperiodic tiling are the mid-point of the centers of the two overlapping hexagons. Edges of the quasiperiodic tiling are obtained by a Delaunay triangulation of the set of reference points. This method is extended to other types of quasiperiodic tilings with other fundamental domains. The use of two square grids leads to the octagonal Ammann-Beenker quasiperiodic tiling (see Figure). This work has been published in Europhysics Letters.
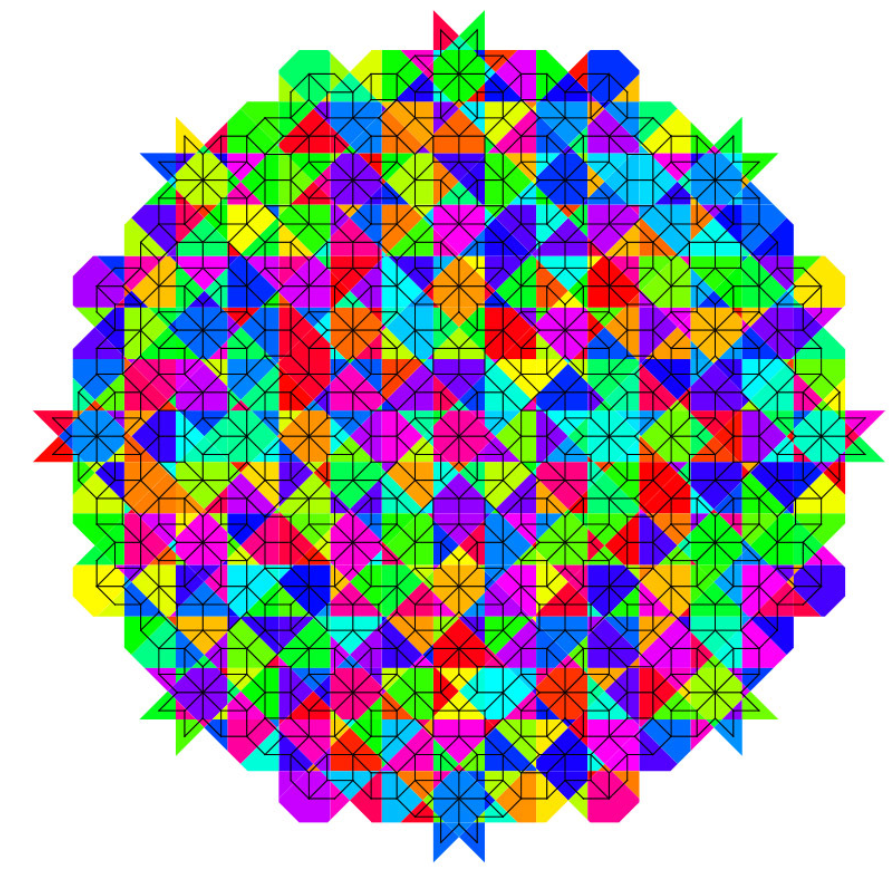
Tessellation of the plane by overlap domains. All domains have a different shape and are plotted with random colors.
Reference
Some examples of quasiperiodic tilings obtained with a simple grid method.
J.-F. Sadoc, M. Impéror-Clerc
EPL, 144, 66002 (2023)
DOI : 10.1209/0295-5075/ad16f3
Contacts
Marianne Impéror-Clerc
Jean-François Sadoc
